My conic likes to hide in boxes

Conics (or conic sections if you like) are very close to my heart. My PhD thesis was about conics and their higher-dimensional relatives, and way back in high school they were one of the bits I particularly loved. So it's no surprise that I get excited each semester when the Maths 1B students study them.

The students, on the other hand, get all worried about it. They wonder how they'll remember the names of all the conics and quadrics, they get all confused about the procedure of figuring out what type of conic the equation represents, and they stress about the fact that drawing them seems so hard. This makes me sad, because they really are very very cool.

One of the things I do to alleviate their pain, is to make the drawing part a little bit easier for them. I created a method of drawing conics in standard form that I like to call "The Method of Boxes". You use the coefficients of the conic to draw a box, and the three different conics live in the box in different ways: the ellipse lives inside the box, the hyperbola lives outside the box, and the parabola lives through the box.

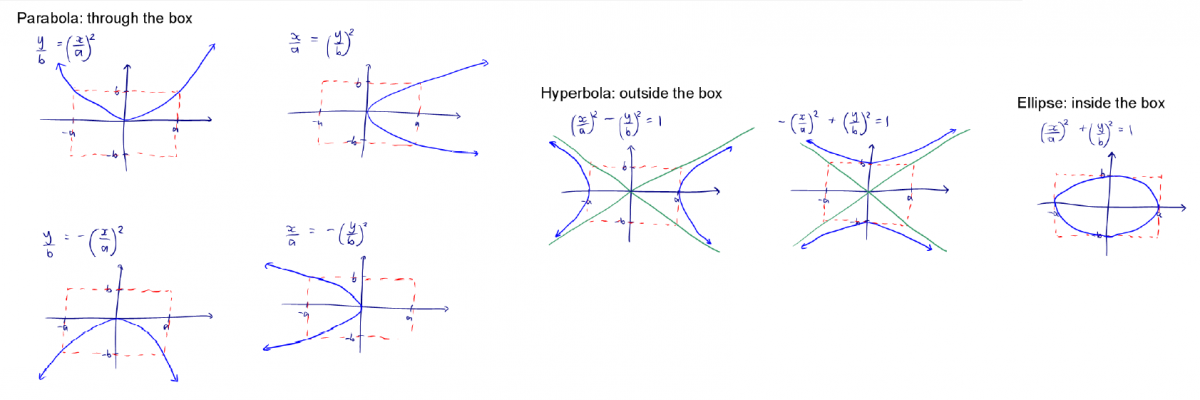
(I've put an explanation of it on YouTube here http://youtu.be/PqBYj1UxJyM.)

It's a beautifully simple method, if I do say so myself, and it has the neat effect of stopping the students worrying about at least one aspect of learning about conics, thus leaving more room for actual learning. But the main thing it does is draw some important connections between the three conics.

The students see the three conics as fundamentally different and so they keep them in their heads separately. The box method literally draws a connection between them – you can draw them all by starting with a box. This connects them all together so they can keep them in their heads in the same place. And the more connections there are, the more you feel you understand.